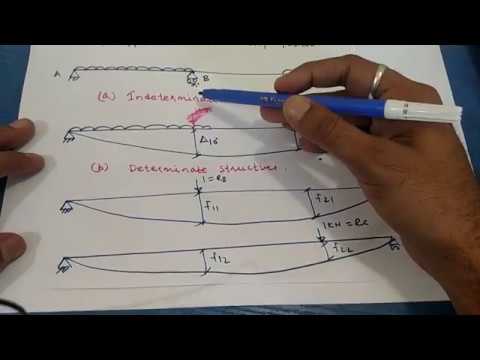
In the realm of structural engineering, achieving harmony between the various components of a structure is crucial for ensuring stability and durability. One key aspect of this harmony lies in the understanding and application of compatibility equations. These equations play a vital role in structural analysis, providing a framework for assessing the compatibility between deformations in different parts of a structure. In this article, we will explore the significance of compatibility equations and their role in maintaining structural integrity.
Defining Compatibility Equations:
Compatibility equations are mathematical expressions used to ensure that the deformations and movements within a structure are consistent and compatible with its overall equilibrium. In simpler terms, these equations help engineers analyze and predict how different parts of a structure will deform or displace in response to applied loads or external forces.
The Link Between Equilibrium and Compatibility:
To understand the importance of compatibility equations, it’s essential to recognize their connection to the principle of equilibrium. Equilibrium ensures that the sum of forces and moments acting on a structure is zero, signifying a state of balance. However, achieving equilibrium alone does not guarantee that a structure will behave as intended.
Compatibility, on the other hand, goes beyond equilibrium by addressing the interdependence of deformations. When loads are applied to a structure, it undergoes deformations that must be compatible with each other to avoid conflicts. Compatibility equations establish the relationships between these deformations. Providing a holistic view of how the entire structure responds to external forces.
Key Components of Compatibility Equations:
- Geometric Compatibility: This aspect focuses on how deformations impact the geometric configuration of the structure. It ensures that deformations do not lead to inconsistencies or conflicts in the overall geometry.
- Material Compatibility: Materials used in construction exhibit specific behaviors under stress and strain. Material compatibility ensures that the deformations within the structure align with the material properties, preventing excessive strains that could compromise integrity.
- Boundary Compatibility: Structures are often anchored or restrained at certain points. Boundary compatibility addresses how deformations are distributed and restrained at these boundaries to maintain stability and prevent undesired movements.
Applications in Structural Analysis:
Compatibility equation find applications in various types of structural analysis, including the assessment of beams, frames, and trusses. By incorporating these equations into analysis procedures, engineers can gain insights into the deformations and movements that occur within a structure, enabling them to make informed decisions about design modifications or reinforcements.
For example, in the analysis of a simple beam subjected to a load, a compatibility equation helps determine how the beam deforms and rotates at different points along its length. Engineers can use this information to assess factors such as bending moments, shear forces, and deflections. Ensuring that the beam’s behavior aligns with design expectations.
Challenges and Considerations:
While compatibility equations provide a powerful tool for structural analysis, their application can pose challenges. Nonlinearities in material behavior, dynamic loading conditions, and complex geometries may require advanced techniques and numerical methods. Engineers must carefully consider these factors to ensure accurate and reliable results in their analyses.
Conclusion:
In the symphony of structural engineering, compatibility equations play a vital role in orchestrating the harmonious interaction between forces, deformations, and materials. Understanding the significance of these equations is essential for engineers seeking to design and analyze structures that not only achieve equilibrium but also maintain compatibility in the face of varying loads and environmental conditions. As technology advances and analytical methods evolve. Compatibility equations remain a foundational element in the pursuit of structural harmony and longevity.